Optimal Phonology (1993)

I. Representatives 

■Prince & Smolensky 1993  (Prince, A. and Smolensky, P. 1993: Optimality Theory: Constraints interaction in generative grammar. MS, Rutgers University and university of Colorado.)

■John McCarthy  1  2

II.  The Rise of OT

III. Optimality Theory

    1. Introduction

    The innovation of Optimality Theory (OT) is to view grammars as a set of ranked violable constraints. These constraints are assumed to be universal, and the difference between child phonology and adult phonology, and between the grammars of different languages, now lies in differences in the ranking of the constraints. Given an input form, a generator, GEN, generates a list of candidates which are assessed by the constraints, the winning candidate being the one which violates not necessarily the fewest, but the lower-ranked constraints. The correct ranking produces the optimal, or most harmonic, form, while other rankings produces less harmonic ones.

    In OT, the candidates are evaluated in a grid known as "tableau", in which constraints are listed in a ranked order to test the candidates. OT introduces constraints that ensure the correct output in not only phonology, but also syllable structure and metrical structure for a language. 

    In short, Optimality Theory machinery is listed: 

    2. Constraints

    There are several main terms and constraints used in OT, which will be briefly introduced in the following passage. 

1. Constraint Ranking: faithfulness

    Surface forms must be faithful to the corresponding lexical forms, that is, surface forms must simply reproduce their lexical counterparts, with no change.

2. Ranking

    The OT equivalent of the ordering rules. A higher-ranked constraint is complied with in preference to a lower-ranked constraint, which is called ranked metaprinciple.

3. Redundancy rule

    This rule can save the corresponding information: the less marked the system, the less information it will need in the lexicon.

4. Sonority constraints

    *M/V=Vowels must  not occupy the syllable margin.

    *N/C=Consonants must not occupy the syllable nucleus.

            (Onset: The constituent that starts the syllable, and precedes the rime.

             Nucleus (N): The most sonorant element of the syllable, typically a vowel.

             Coda: The consonant that follows the nucleus, and that joins it to make up the rime.

             Rime (R): An immediate constituent of the syllable placed after the onset and made up of the nucleus and the coda.)

5. Dependency (DEP)

    The constraint that blocks epenthesis, which require every surface segment to have a lexical correspondent, on which it therefore "depend."

6. Fatal violation

    A violation disqualified a candidates vis-à-vis some other candidate after the corresponding asterisk conventionally marked by an exclamation mark ("!").

7. Maximality (MAX)

    The constraint that is responsible for the maximal mapping of underlying forms onto the surface, which is called parse in old OT.

8. Strict layer hypothesis

    Each phonological domain contains precisely one or more phonological domains of the rank immediately below.

9. Complex

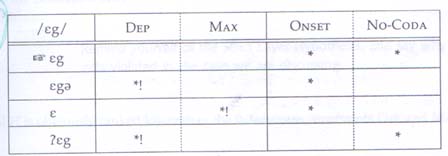
    So far, some of the terms in OT listed above can be used in a tableau for evaluation, here is an example from the reference (Roca & Johnson, 2000) In a tableau, constraint violations are signaled with an asterisk, while constraint compliances are left blank.  In the tableau below,  for example, Dep and Max are in the same tanking, while Onset and No-Coda are in the same ranking, which is lower than Dep and Max. Dotted line represents the same level ranking constrains. And a hand mark is the item that win out of the evaluation. We can see how constraints work in OT to select the most harmonic form. 

    A simpler example:

    while a more complex one:

　Another example demonstrates syllable complexities:

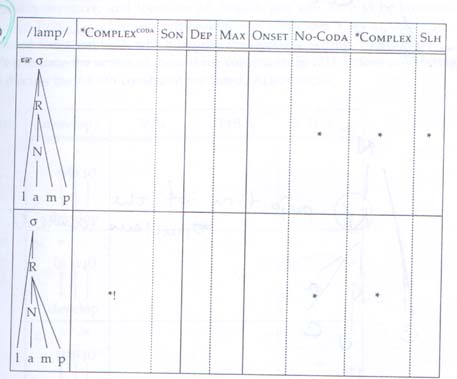
There are further concepts of OT that are not listed here, such as Basic metrical structure, Extrametricality, Quantity sensitivity, Secondary footing, Corresponding constraints, Cyclic effect, Word formation through truncation, and OT morphology. Further reading is accessible.

IV. Relative websites

■ROA is a co style="line-height: 150%;e, downloadable papers and dissertations on generative linguistics, esp. syntax and phonology, under Optimality Theory

■Optimality Theory 0. Introduction As you have seen from other pages, the history of phonology starts with rules which are applied in a specific order to a UR to produce the correct surface form. Besid

■Hopkins Optimality Theory Workshop / University of Maryland Mayfest 1997 May 9-12, 1997

■Optimality Theory :Dekkers -Site Search OUP Web Sites English Language Teaching Journals OED Oxford World's Classics Resources for Schools Dictionaries New DNB Popular Science Best TextbooksElectronic PublishingEmail NewsGeneral

■Optimality Theory Syntax Project Institut fur Linguistik/Anglistik Institut fur Linguistik/Germanistik University of Stuttgart Postfach 10 60 37 D-70049 Stuttgart GERMANY (First) WORKSHOP ON OPTIMAL

■Seminar on Optimality Theory - Contents: Schedule Handouts Readings OT-Related Links University of Rochester Seminar on Optimality Theory Spring, 2000 Ed Keer, ekeer@ling.rochester.edu Katherine Crosswhite, crosswhi@ling.rochester

■Computation and Learning in Optimality Theory Dicky Gilbers, Gertjan van Noord. H.15.055 op woensdag van 11-13 uur. Daarnaast de fonologie-3 colleges op woensdag 15-17 uur op 20 oktober en 27 oktober

■Variation and Change in Optimality Theory

Paper Index Page Variation and Change in Optimality Theory Authors: Arto Tapani Anttila --> e-mail Young-mee Cho --> e-mail Keywords: Historical Linguistics Morphology Phonology linking/intrusive-r analogy statistical regularities partial...

■UCI

■MIT Optimality ConferenceIT Optimality Conference"MsoNormal" align="center" style="line-height: 150%; margin-top: 1">